
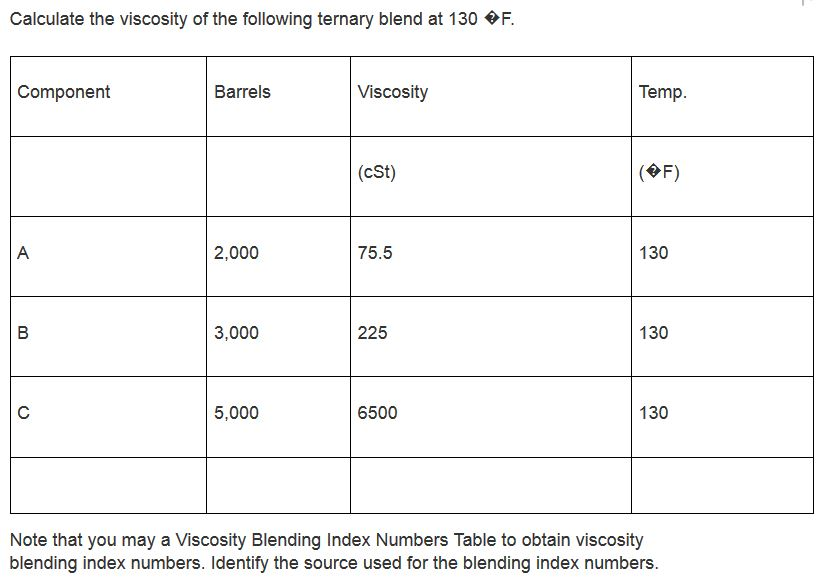
Given M w and M n the standard deviation of a distribution of molecular weights can be determined: The polydispersity index, M w/M n, is the ratio of the second moment to the square of the first moment about the origin. Or the ratio of the second to the first moment about the origin. The weight average molecular weight (mass average), M w, is given by: The number average molecular weight, M n is the same as the first moment about the origin or the mean.

These parameters can be described in terms of the more broadly used statistical description of moments given above. The number average, weight average, z-average and viscosity average. In polymer science it is the convention to define several special parameters to describe molecular weight distributions. The variance, s 2, is the second moment (k = 2) about the mean (x s = m ). For example, the mean, m, is the first moment (k = 1) about the origin (x s = 0). N i/N, x s is basis for the moment and k is the order of the moment. Where f i is the fraction of all measurements which have the value x i, i.e. The k'th moment of a distribution about a value, x s, is given by:
More complicated continuous distribution functions will involve higher moments of the distribution. The simplest description of a unimodal (one peak) symmetric distribution (same on both sides of the peak) will involve two parameters, the mean, m and the standard deviation, s. Such a distribution of molecular weights can be described by statistics in terms of moments. The distribution shown above is bimodal (two identifiable peaks) and skewed (neither of the peaks is a symmetric about their means and the entire bimodal distribution is not symmetric about the mean.
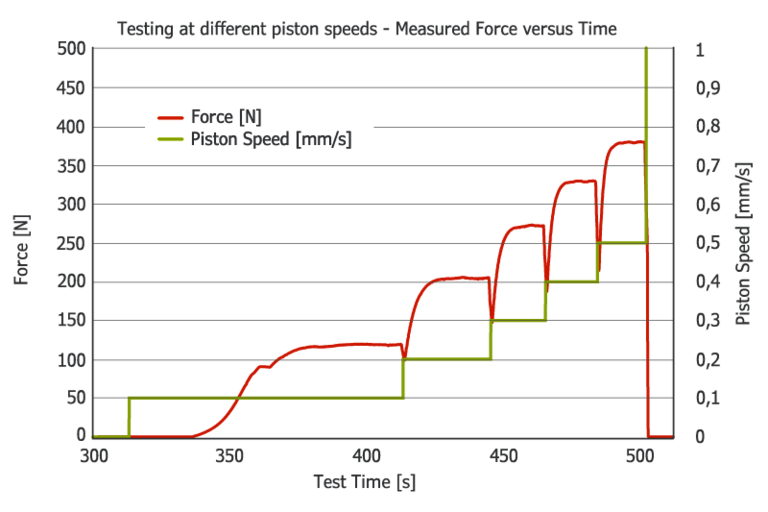
Consider a plot of the fraction of a plastic sample with a specified molecular weight versus molecular weight as show below. The features of this distribution have dramatic effects on the processability (viscosity) and properties (miscibility, strength and modulus) of plastics.
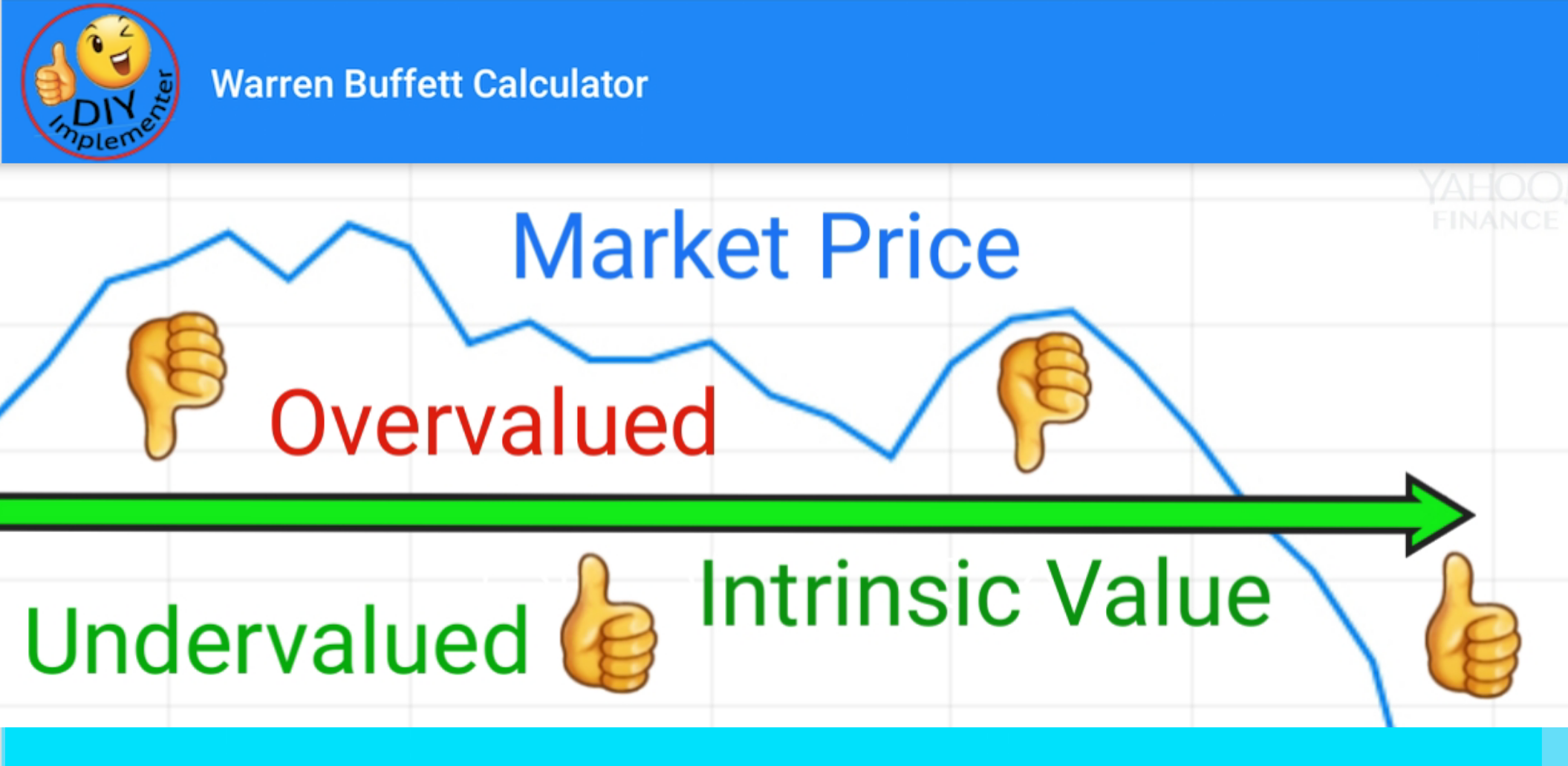
From typical industrial synthesis a fairly broad distribution in molecular weight results. The distinguishing feature of commercial polymers is that they have molecular weights far in excess of the entanglement molecular weight of about 10,000 g/mole. Download this page: =>MolecularWeight.pdf


 0 kommentar(er)
0 kommentar(er)
